Lets define the following events:
$P$ : Uppermost link is working.
$Q$: Middle link is working.
$R$: Lowermost link is working.
$W$ : Terminals $A$ & $B$ are connected.
From the given information, we can calculate the probabilities of events $P$, $Q$ and $R$ as follow:
(as I already consumed letter (capital) $P$ denoting an event so here by I will use letter (small) $p$ to denote probabilities,
e.g. $p\left ( X \right )$ denotes probability of happening of event $X$).
$\qquad p\left ( P \right ) = \dfrac{4}{5} \times \dfrac{4}{5} = \dfrac{16}{25}$,
$\Rightarrow\; p\left ( \widehat{P} \right ) = 1 - \dfrac{16}{25} = \dfrac{9}{25}$,
$\qquad p\left ( Q \right ) = \dfrac{2}{3} $,
$\Rightarrow\; p\left ( \widehat{Q} \right ) = \dfrac{1}{3} $,
$\qquad p\left ( R \right ) = \dfrac{3}{4} \times \dfrac{3}{4} = \dfrac{9}{16}$,
$\Rightarrow\; p\left ( \widehat{R} \right ) = 1 - \dfrac{9}{16} = \dfrac{7}{16}$.
The situation here can be represented using the following Venn Diagram:
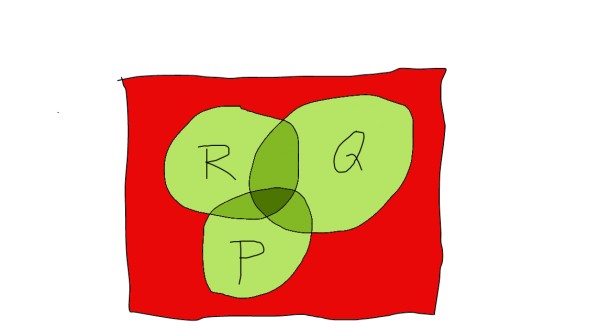
Here Red region denotes the event $\widehat{W}$ in which $A \& B$ are disconnected.
Different gradients of Green colour represent the $W$ in which terminals $A \& B$ are connected.
We have to find $p\left ( W \right )$
Events $W$ and $\widehat{W}$ are mutually exclusive & totally exhaustive,
since either terminals $A$ and $B$ will be connected or they will be disconnected.
So we can write $p\left ( W \right )$ in terms of $p\left ( \widehat{W} \right )$ as follow:
$ p\left ( W \right )= 1 - p\left ( \widehat{W} \right )$.
Also it can be seen that
$ p\left ( \widehat{W} \right )= p\left ( \widehat{P} \right ) \cap \left ( \widehat{Q} \right ) \cap \left ( \widehat{R} \right )$ where $\widehat{P}$, $\widehat{Q}$ and $\widehat{R}$ are independent events.
That is terminals $A$ & $B$ will be disconnected only when all of the links will fail simultaneously.
Using independence we can write,
$p\left ( \widehat{W} \right ) = p\left ( W \right )= p\left ( \widehat{P} \right ) \times \left ( \widehat{Q} \right ) \times \left ( \widehat{R} \right )$,
$\Rightarrow p\left ( \widehat{W} \right )= \dfrac{9}{25} \times \dfrac{1}{3} \times \dfrac{7}{16} = \dfrac{21}{400}$
$\Rightarrow p\left ( W \right )= 1 - \dfrac{21}{400} = \dfrac{379}{400}$.
Correct Answer: $B$