TIFR CSE 2014 | Part A | Question: 17

Question

A fair dice (with faces numbered $1, . . . , 6$) is independently rolled repeatedly. Let $X$ denote the number of rolls till an even number is seen and let $Y$ denote the number of rolls till $3$ is seen. Evaluate $E(Y |X = 2)$.

• A. $6\frac{5}{6}$
• B. $6$
• C. $5\frac{1}{2}$
• D. $6\frac{1}{3}$
• E. $5\frac{2}{3}$

Comments

5.66

---

Can you explain your approach?

Answer 1

Answer is (E)

$X$: The value of $X$ denotes the number of rolls till an even number is seen.

$Y$:  The value of $Y$ denotes the number of rolls till a $3$ is seen.

For example:

$X = 2$ implies an even number first time occurred on second roll, or outcome of the first roll is odd & outcome of the second roll is even.

$Y = 4$ implies $3$ appeared for first time in the $4^{th}$ die roll.

Ranges of Random Variables $X$ & $Y$

$X:\{ 1, 2, 3,\ldots ,\infty \}$

$Y:\{1, 2, 3,\ldots,\infty \}$ 

$E[Y\mid X = 2]:$ Expected number of rolls till a $3$ is seen given that an even number appeared for the first time in the second roll.

It is sure that $3$ cannot appear on $2^{nd}$ toss, i.e. $P[Y =2\mid X =2] = 0$ and henceforth $E[Y =2\mid X =2] = 0.$

Now, there are two cases possible:

Case 1: 3 appears on the first toss given that outcome of first toss is odd.

i.e., $E[Y = 1\mid X =2]$

Here we need not to concern about outcomes of rolls other than the first roll.

Probability of getting $3$ in first toss given that o/c of the first toss is odd $=P(Y = 1\mid  X = 2)   =\dfrac{1}{3} = 0.33$

So, Expectation $ E[Y = 1\mid X = 2] = y\times P(Y = 1\mid X = 2) =1 \times 0.33 = 0.33$

Case 2: 3 appears on any toss after the second toss given that outcome of first toss is odd, & that of second toss is even

$P[Y = y \mid X = 2]$ = given that $1^{st}$ roll is an odd number and  $2{nd}$ roll is an even number, Probability that out of $y$ rolls,

None of the first $(y – 1)$ roll’s outcome is $3$ &

Outcome of the $y^{th}$ roll is $3$.

So $P[Y = y \mid X = 2] $
$\qquad = \left(\dfrac{2}{3}\right)(\text{for first o/c odd but not } 3)$
$\qquad \times \left(\dfrac{5}{6}\right)^{(y – 3)}( \text{for not getting a} \;3\; from\;3^{rd} \;to\;0 (y – 1)^{th} rolls)$
$\qquad \times \left(\dfrac{1}{6}\right)(\text{for }y^{th} \text{o/c to be}\; 3).$

$P[Y = y \mid X = 2] = \left(\dfrac{2}{3}\right)\times \left(\dfrac{5}{6}\right)^{(y – 3)}\times \left(\dfrac{1}{6}\right)$

So $E[Y = y \mid X = 2]=$ Summation from $y = 3 \text{ to infinity}(y\times P(Y = y \mid X = 2)) = {5.33}$ (where $y\geq 3$)

This summation will give sum of all the expectations from $Y = 3 \text{ to infinity}.$

Now:

Net Expectation is given as:

$E[ Y = y\mid X =2] = E[ Y = 1\mid X = 2] + E[ Y = 2\mid X =2] + E[ Y = y’\mid X =2]$ where $y’\geq 3.$

Putting all the values,

$E[ Y = y\mid X =2] = 0.33 + 0 + 5.33$

So, $E[ Y = y\mid X =2] = 5.66 =\dfrac{17}{3}.$

Comments

can u explain for p[Y=y|X=2]  where y ranges from 3 to infinity

(2/3) (for first outcome to be odd but not 3) * (1/2)(for second outcome to be even number) *(5/6)^(y-3)(for not getting 3)(1/6) (when we get  3 number in 3rd roll)  why we are not multiplying prob of second outcome to be even number here

---

By definition, P[Y = y | X = 2] denotes the probability of getting a 3 on the die for the first time on the yth roll, given the outcome of the second roll is an even number and the outcome of the first roll was not an even number.

We certainly know that the outcome of the 2nd roll has to be an even number so we need not multiply 1/2.The Probability of getting an even number on the second roll is 1. Also, the probability of not getting an even number on the first roll or equivalently the probability of getting an odd number on the first roll is 1.

P[Y=1|X=2] = Given the outcome of the first roll is not an even number, the probability that the outcome is 3 = {3}/{all odd numbers} = 1/3.

P[Y=2|X=2] = Given the outcome of the first roll is neither an even number nor 3 and given the outcome of the second roll is an even number, the probability that the outcome of the second roll is 3 = ({all odd numbers except 3}/{all odd numbers}) x 0 = 0 as we know that the outcome of the second roll is an even number and thus it can not be 3 at all.

P[Y=3|X=3] = Given the outcome of the first roll is neither an even number nor 3 and given the outcome of the second roll is an even number, the probability that the outcome of the third roll is 3 = ({all odd numbers except 3}/{all odd numbers}) x ({3}/{all numbers}) = (2/3) x (1/6) = 1/9.

---

okk got it t

---

Well explained.Thanks:)

---

Can you please explain how you did the summation part:$"E[Y=y∣X=2]= Summation\:from\:y=3\: to\: infinity\:(y×P(Y=y∣X=2))=5.33 \: (where \:y≥3)"$.

Is the summation = $(1/9)\sum_{i=3}^{\infty} i*(5/6)^{i-3}$?If yes, how to solve this?

---

I am getting final answer as 5.99 correct if I am wrong you can solve this by arithmetic geometric expression AGP getting answers as 5.66

---

Can someone explain the summation?

---

$Summation\;(S)$

$S=\left(\frac{2}{3}\right)\sum_{y=3}^\infty y\left(\frac{5}{6}\right)^{y-3}\left(\frac{1}{6}\right)$

$9S=\sum_{y=3}^\infty y\left(\frac{5}{6}\right)^{y-3}$

$9S=3+4\left(\frac{5}{6}\right)+5\left(\frac{5}{6}\right)^2+\ldots\to\color{green}{(1)}$

$\color{green}{(1)-(1)}\times\frac{5}{6}$

$9S\left(1-\frac{5}{6}\right)=3+\left(\frac{5}{6}\right)+\left(\frac{5}{6}\right)^2+\left(\frac{5}{6}\right)^3+\ldots$

$\frac{3S}{2}=3+\left(\frac{5/6}{1-5/6}\right)$

$\frac{3S}{2}=8$

$S=16/3=5.33$

Answer 2

Wrong Solution for the unedited question.

Unedited question:

A fair die(with face numbered 1, 2, ....., 6) is independently rolled repeatedly.Let X denote the number is seen & let Y denote the number of rolls till 3 is seen. Evaluate E(Y|X =2)?

A) 41/6

B) 6

C) 11/2

D) 19/3

E) 17/3.

Solution:

I am getting 6 as an APPROXIMATED answer.

This is just an okay method, most probably there must be better & more intuitive solutions to this problem.

X & Y here are random variables. They will contain some values from their ranges of allowed values.

X : The value of X is seen.

Y:  The value of Y denotes the number of rolls till a 3 is seen.

For example:

X = 2 implies 2 is seen,

Y = 4 implies 3 appeared for first time in the 4^th die roll.

Ranges of Random Variables X & Y

X : { 1, 2, 3, 4, 5, 6}

Y: {$1, 2, 3, \ldots\infty$}  

-----------------------------------------------------------------------------------------------------------------

E[Y | X = 2]: the expected (or average) number of rolls till a 3 is seen, given that 2 is seen.

In other words, average number of rolls till 3 appears for the first time in repeated die rolls, given that 2 has been already appeared at least once.

From the definition of Expectation:

$\displaystyle E[Y = y|X = 2] =\sum_{y=1}^{\infty}
{y * P(Y = y | X = 2)}$;

Where  is the probability that 3 appeared for the first time in y^th die roll, given that 2 already has been appeared at least once in the first (y - 1) die rolls.

P( Y = 1|X = 2) = 0,  since there is only one die roll, 3 can't appear after 2.

-----------------------------------------------------------------------------------------------------------

Calculation of P(Y = y|X = 2):

From the definition of conditional probability, for y die rolls:

$P(Y = y | X = 2) = \frac{(P(Y = y) \cap P(X = 2))}{P(X = 2)}$

i.e. $P(Y = y | X = 2) = \frac{\textrm{Probability of first appearance of 3 in the yth roll and  AT LEAST ONE  appearance of 2 in the first (y-1) die rolls}}{\textrm{Probability of at least one appearance of 2 in y die rolls}}$

--------------------------------------------------------------------------------------------------------------

Calculating Denominator: (Probability of at least one appearance of 2 in y die rolls)

$P(Denominator) = \frac{\textrm{Outcomes in which 2 is appearing at least once}}{\textrm{All possible outcomes}}$

$P(Denominator) = \frac{(C_{1}^{y} 6^{(y – 1)} – (y – 1))}{6^y}$

$\textrm{How } (C_{1}^{y} *6^{(y – 1)} – (y – 1))$

Out of y places chose any one place for 2(yC1), in the remaining (y – 1) places any of the 6 outcomes can be placed minus (y -1) repetitions.

----------------------------------------------------------------------------------------------------------------

Calculating Numerator: (Probability of first appearance of 3 in the y^th roll and AT LEAST ONE  appearance of 2 in the first (y-1) die rolls)

$P(Numerator) = \frac{\textrm{Outcomes in which 2 is appearing at least once in first (y-1) rolls and 3 is appearing in the last roll}}{\textrm{All possible outcomes}}$                                    

 $P(Numerator) = \frac{C_{1}^{(y - 1)}5^{(y -2)} – (y-2)}{6^y}$

$\textrm{How } C_{1}^{(y - 1)}5^{(y -2)}– (y-2)$

3 is fixed on the y^th place, out of remaining (y – 1) places chose a place for 2 ((y-1)C1) and fill any of the 5 Outcomes(all possible outcomes EXCEPT 3) in the remaining (y-2) places minus (y -2) repetitions.

---------------------------------------------------------------------------------------------------------------

Now,

$P(Y = y | X =2) = \frac{\textrm{Probability of first appearance of 3 in the y^th roll and AT LEAST ONE  appearance of 2 in the first (y-1) die rolls}}{\textrm{Probability of at least one appearance of 2 in y die rolls}}$

 $P(Y = y | X = 2) = \frac{P(Numerator)}{P(Denominator)}$

On putting the values & simplification,

$P(Y = y | X = 2) = \frac{((y-1)5^{(y-2)}-y+2)}{(y6^{(y-1)}-y+1)}$

By putting the value calculated above, Expectation E[Y | X = 2], can be given as follow:

$ \displaystyle E[Y = y|X = 2] = \sum_{y=2}^{\infty}
y*\frac{((y-1)5^{(y-2)}-y+2)}{(y*6^{(y-1)}-y+1)}$

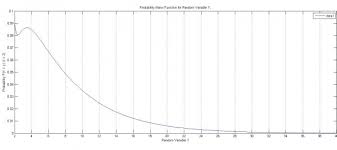
I don’t know how to converge the  Probability Mass Function(P(Y = y|X =2) so I calculated expectation by varying y from 2 to 120(Not Infinity)using fx991ES calculator & got 5.981334741.

I guess, Expectation to be 6.

Here is the plot for a portion of the Probability Mass Function(P(Y = y|X  = 2) in which y is varying from 2 to 40, & weighted average of such a complete graph will give the expectation.

Comments

Thank u anurag for ur explanation.....

Can't we use this approach

No of String with last no is 3 and atleast one 2 =5^(y-1)-4^(y-1)

Here total string possible is 5^(y-1) and I am subtracting all string with no 2 at any of this y-1 pos we are restricted to use 3 already and now 2 also means 4^(y-1)

Total no of string with atleast 2 appear once is 6^(y) - 5^(y)

So PMF will be {y (5^(y-1)- 4^(y-1))/(6^y-5^y)} please correct me if I am thinking in wrong direction

---

Exactly! you are right Saurav.

I got the same PMF after correction,

& even the blue line in my previous comment is the plot for that PMF.

& this PMF will give the value of expectation for that unedited question near about 5.75

---

I am getting final answer as 5.99 correct if I am wrong you can solve this by arithmetic geometric expression AGP getting answers as 5.66 so final answer hai approximated to 6 hai

Answer 3

$X = \# \text{Rolls till even number is seen}$

$Y = \# \text{Rolls till number 3 shows up}$

We need to find $\mathbb{E}(Y \ | \ X = 2)$

---

$$\begin{align}
\mathbb{E}(Y \ | \ X = 2) &= \sum_{y=0}^{\infty} yP(Y=y \ | \ X=2) \\ 
&= \sum_{y=0}^{\infty} y \dfrac{P(Y=y, X=2)}{P(X=2)} = \sum_{y=0}^{\infty} y \dfrac{P(Y=y, X=2)}{\underbrace{\frac{3}{6}}_{\text{No even number}}.\underbrace{\frac{3}{6}}_{\text{| Only even number}}}
\end{align}$$

$\underline{\textbf{Observation(s)}}$

$P(Y=2, X=2) = 0$, since at second roll we having even number as $X=2$, and $3$ is odd, hence $3$ can't show up on the second roll as $Y=2$ is indicating. 

---

$$\begin{align}
\mathbb{E}(Y \ | \ X=2) &= 4 \Big[0.P(Y=0, X=2) + 1.P(Y=1, X=2) + 3.P(Y=3, X=2) + \dots\Big]\\
&=  4\Big[1.\underbrace{\frac{1}{6}}_{\{3\}}.\underbrace{\frac{3}{6}}_{\{2,4,6\}} + 3.\underbrace{\frac{2}{6}}_{\{1,3,5\}-\{3\}}.\underbrace{\frac{3}{6}}_{\{2,4,6\}}.\underbrace{\frac{1}{6}}_{\{3\}} + 4.\frac{2}{6}.\frac{3}{6}.\underbrace{\frac{5}{6}}_{\substack{\{1,2,4,5,6\}}}.\frac{1}{6} + 5.\frac{2}{6}\frac{3}{6}\frac{5}{6}\frac{5}{6}\frac{1}{6}+\dots\Big]\\
&= 4 \Big[1.\frac{1}{12} + 3.\frac{1}{36} + 4.\frac{1}{36}.\frac{5}{6} + 5.\frac{1}{36}.\Big(\frac{5}{6}\Big)^2 + 6.\frac{1}{36}.\Big(\frac{5}{6}\Big)^3 + \dots\Big] \\ 
&= 4 \Bigg[\frac{1}{12} + \frac{1}{36} \underbrace{\Big[3 + 4.\Big(\frac{5}{6}\Big)^1 + 5.\Big(\frac{5}{6}\Big)^2 + 6.\Big(\frac{5}{6}\Big)^3 + \dots\Big]}_{AGP}\Bigg]\\
&= 4 \Bigg[\frac{1}{12} + \frac{1}{36}\Big[48\Big]\Bigg]\\
&= \frac{17}{3} = 5\frac{2}{3}
\end{align}$$

$\textbf{Option (E) is correct}$

---

$\underline{\textbf{AGP Solution}}$

Suppose you have a series $a, (a+d)r, (a+2d)r^2, (a+3d)r^3, \dots$

Then sum of this series is given by $\dfrac{a}{1-r} + \dfrac{dr}{(1-r)^2}$

Above we are having $a=3, d=1, r=\frac{5}{6}$